$\mathbb{R}^3$の極小曲面
Weierstrassの表現公式
$M$をRiemann面, $(g,\eta)$を$M$上の有理型関数と正則1次微分の組で, \[ \big(1+|g|^2\big)^2\eta\overline{\eta} \] が$M$上のRiemann計量を定めるものとする. このとき \[ f(p)=\mathrm{Re}\int_{p_0}^p\big(1-g^2,\,i(1+g^2),\,2g\big)\eta \] は$M$から$\mathbb{R}^3$への共形極小はめ込みを与える. ただし$p_0$は$M$上の定点である. $f$の定義に線積分を用いているので, $f$は一般に$M$上多価である.
例 (18世紀)
例 (19世紀)
- Scherk's first surface (doubly periodic).
- Scherk's second surface (associate surface of catenoid).
- Scherk's third surface (branched).
- Scherk's fourth surface (branched, singly periodic).
- Scherk's fifth surface (singly periodic).
- Associste family of Scherk's first and fifth surfaces
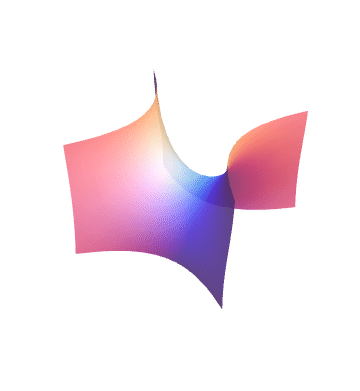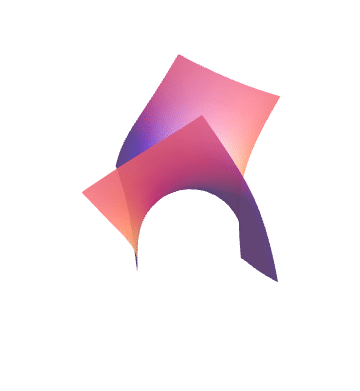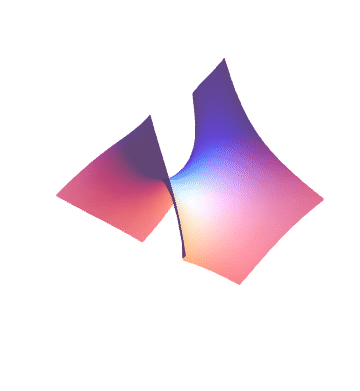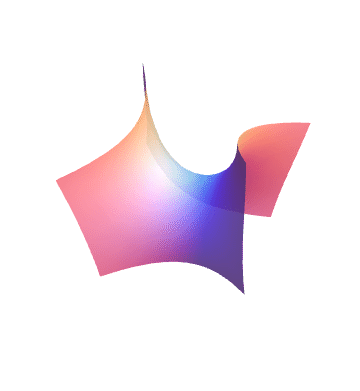
- Enneper surface.
- Riemann's minimal surface (singly periodic).
- Schwarz primitive family (triply periodic).
例 (20世紀)
向き付け不可能
- Moebius strip by Meeks and Oliveira.
- Another Moebius strip (cf. Meeks-Weber).
- Kusner's projective plane with $2n+1$ ends.
- López' Klein bottle with one end and its generalization by López and Martín.
非周期的
- Jorge-Meeks $n$-noid.
- Jorge-Meeks $n$-noid plus 2 horizontal ends.
- Xu's surface with icosahedral symmetry.
- A planar end between two catenoid ends (finite Riemann 1).
- Two planar ends between two catenoid ends (finite Riemann 2).
- Three planar ends between two catenoid ends (finite Riemann 3).
- Chen-Gackstatter surface family.
- Costa-Hoffman-Meeks surface of genus $k$.
- Double Enneper of genus $k$.
単周期的
二重周期的
三重周期的
例 (21世紀)
- Two-ended surfaces with least total absolute curvature by Fujimori and Shoda.
- Schwarz P with Neovius handles by Fujimori and Weber (triply periodic).
- 随時追加します.
謝辞
このサイトを作成するにあたり, Matthias Weber氏のMinimal Surface MuseumやMinimal Surface Repositoryを参考にしました. また, 極小曲面の描画に関してはWeber氏以外にもPeter Connor氏, 小林真平氏, 中村英史氏, Wayne Rossman氏, Seong-Deog Yang氏から有益なご意見をいただきました. ここに記して感謝の意を表します.
Links
- Minimal Surface Repository (Matthias Weber氏)
- Gallery of Minimal Surfaces (Sisto Baldo氏)
- 旧極小曲面ギャラリー