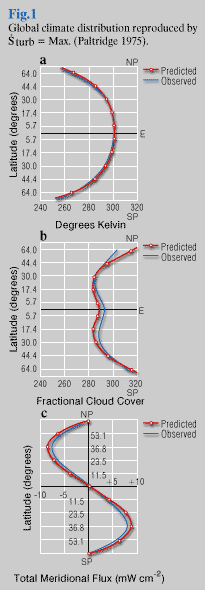
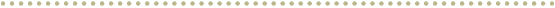It has long been suspected that the long-term mean state of the earth's climate may represent a state of maximum entropy increase. Paltridge (1975, 1978) first suggested that the global distribution of the present climate (e.g., mean air temperature, cloud amount, and horizontal heat transport) is reproducible by a constraint of the maximum rate of entropy increase by the turbulent heat transport in the atmosphere and ocean.
Fig.1 shows such an example (Paltridge, 1975). Several researchers checked Paltridge's work and got essentially the same result (e.g., Grassl, 1981; Noda and Tokioka, 1983). His suggestion was criticised by Essex (1984), however, since a predominant contribution to entropy increase in the planet is due to direct absorption of solar radiation at the earth's surface. We can write the total rate as a sum of the entropy increase rate by the turbulent contribution and that by the absorption:
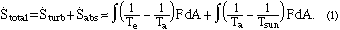
A rough estimation can show that the latter contribution (
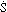 abs
abs) is more than ten times greater than the former one (
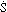 turb
turb). Then, there appears to be no reason for
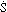 turb
turb to be a maximum independent of
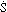 abs
abs. Since then, the radiation problem has been a central objection to the Paltridge work (e.g., Stephens and O'Blien, 1993; Li and Chylek, 1994; Pujol and Llebot, 1999).
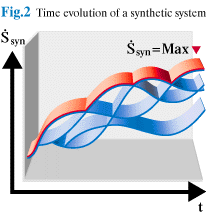
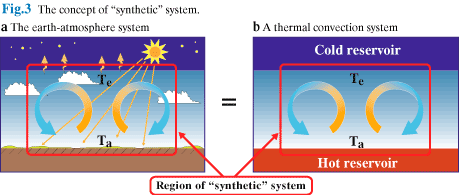
Recently, we have revised the Paltridge work in the light of a thermodynamic variational concept, an extension of an idea of Sawada (1981). It states that entropy of thermal reservoirs connected through a non-linear system, in which materials interact mutually, will increase along a path of evolution with a maximum rate of entropy increase, among a manifold of allowed paths (Fig.2). The region of the system connected by the mutual interaction of the non-linear system is called the "synthetic" system. In the case of the turbulent heat transport in the earth-atmosphere system, the synthetic region is limited to the non-linear fluid system, the surface of the earth, and the top of the atmosphere (see Fig.3(a)). The radiative transport process is excluded form the synthetic part, since it is a directional transport process and not a mutual interaction. Intuitively, it is clear that, for the fluid system, the solar radiation works just as heat source at the surface with the absorption temperature of Ta, and the fluid system does not mind the entropy increase by the absorption of solar radiation. Then, the situation will be the same as a convection system located between a hot reservoir (Ta) and a cold reservoir (Te) (Fig.3(b)). The entropy increase rate for the synthetic system is, then, identical to that due to the turbulent heat transport process, which should be a maximum:
if a steady-state for the fluid system can be assumed. We applied the thermodynamic constraint (2) to the turbulent heat transport process in the atmosphere, and thereby reproduced vertical distributions of air temperature and energy fluxes that resemble those of the present earth (Ozawa and Ohmura, 1997). Thus, it is found that Paltridge's suggestion on the maximum entropy increase in the earth's system, as well as Malkus-Howard's suggestion on maximum heat transport in thermal convection (F=Max.), is consistent with the thermodynamic concept, provided that the maximum constraint is applied to the synthetic system only (
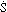 syn
syn = Max.), regardless of the radiation term (
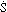 abs
abs).
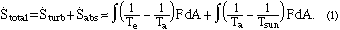
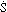 abs) is more than ten times greater than the former one (
abs) is more than ten times greater than the former one (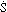 turb). Then, there appears to be no reason for
turb). Then, there appears to be no reason for 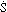 turb to be a maximum independent of
turb to be a maximum independent of 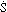 abs. Since then, the radiation problem has been a central objection to the Paltridge work (e.g., Stephens and O'Blien, 1993; Li and Chylek, 1994; Pujol and Llebot, 1999).
abs. Since then, the radiation problem has been a central objection to the Paltridge work (e.g., Stephens and O'Blien, 1993; Li and Chylek, 1994; Pujol and Llebot, 1999).