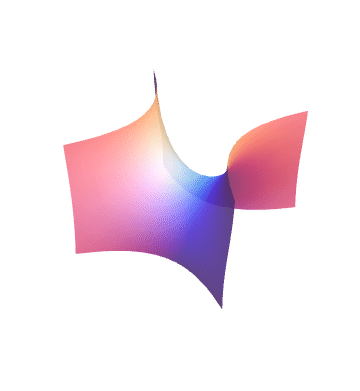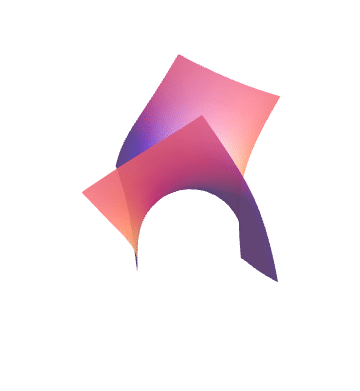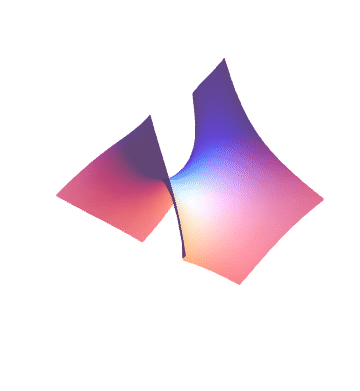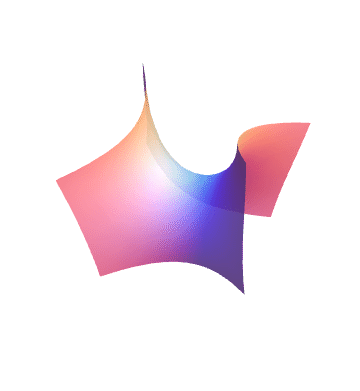
Minimal Surfaces in $\mathbb{R}^3$
Weierstrass Representation
Let $M$ be a Riemann surface and $(g,\eta)$ a pair of meromorphic function and holomorphic 1-form on $M$ such that \[ \big(1+|g|^2\big)^2\eta\overline{\eta} \] gives a Riemannian metric on $M$. Then \[ f(p)=\mathrm{Re}\int_{p_0}^p\big(1-g^2,\,i(1+g^2),\,2g\big)\eta \] gives a conformal minimal immersion (possibly multi-valued on $M$) into $\mathbb{R}^3$, where $p_0$ is a fixed point on $M$.
Examples (18th century)
Examples (19th century)
- Scherk's first surface (doubly periodic).
- Scherk's second surface (associate surface of catenoid).
- Scherk's third surface (branched).
- Scherk's fourth surface (branched, singly periodic).
- Scherk's fifth surface (singly periodic).
- Associste family of Scherk's first and fifth surfaces
- Enneper surface.
- Riemann's minimal surface (singly periodic).
- Schwarz primitive family (triply periodic).
Examples (20th century)
Non-orientable
- Moebius strip by Meeks and Oliveira.
- Another Moebius strip (cf. Meeks-Weber).
- Kusner's projective plane with $2n+1$ ends.
- López' Klein bottle with one end and its generalization by López and Martín.
Non-periodic
- Jorge-Meeks $n$-noid.
- Jorge-Meeks $n$-noid plus 2 horizontal ends.
- Xu's surface with icosahedral symmetry.
- A planar end between two catenoid ends (finite Riemann 1).
- Two planar ends between two catenoid ends (finite Riemann 2).
- Three planar ends between two catenoid ends (finite Riemann 3).
- Chen-Gackstatter surface family.
- Costa-Hoffman-Meeks surface of genus $k$.
- Double Enneper of genus $k$.
Singly periodic
Doubly periodic
Triply periodic
Examples (21st century)
- Two-ended surfaces with least total absolute curvature by Fujimori and Shoda.
- Schwarz P with Neovius handles by Fujimori and Weber (triply periodic).
- to be continued...
Acknowledgements
This site is inspired by Minimal Surface Museum and Minimal Surface Repository maintained by Matthias Weber. I thank him, and I also thank Peter Connor, Shimpei Kobayashi, Fusashi Nakamura, Wayne Rossman, and Seong-Deog Yang for valuable comments about drawing minimal surfaces.
Links
- Minimal Surface Repository by Matthias Weber
- Gallery of Minimal Surfaces by Sisto Baldo
- My old minimal surface gallery